Is 15 A Rational Number

The 101 to mastering any subject is the knowledge of its lexicon. The way nosotros combine the letters of the alphabet to make a word and so arrange words in sentences to convey our thoughts is similar a natural instinct to us but because we have the cognition of the English alphabet. And so, what should be the 101 for making maths a natural instinct? The knowledge of its rudimentary unit: numbers. We teach counting using whole numbers, but i of the most important aspect of the mathematical numbers system is rational numbers, or but put, numbers between numbers.

What Are Rational Numbers?

Rational numbers are numbers that can exist written in the course pq, where p and q are integers and q≠0. The difference between rational numbers and fractions lies in the fact that fractions cannot have negative numerator or denominator. Hence, the numerator and denominator of a fraction are whole numbers (denominator ≠ 0), whereas in the example of rational numbers, the numerator and denominator are integers.

Examples of Rational Numbers

$\frac{4}{v}$, $\frac{-10}{15}$, $\frac{9}{-17}$, $\frac{-ii}{-7}$

Null is a rational number as information technology tin can be written as $\frac{0}{10}$, $\frac{0}{2}$, $\frac{0}{-15}$, $\frac{0}{27}$, etc.

And then, zero tin be expressed equally a fraction with a non-cypher denominator.

Every natural number is a rational number.

1 = $\frac{i}{i}$, five = $\frac{5}{1}$, 8 = $\frac{8}{1}$

So, every natural number tin be expressed as a fraction with 1 as the denominator.

Every integer number is a rational number.

-7 = $\frac{-7}{1}$, -xiii = $\frac{-13}{ane}$

And so, every integer number can be expressed every bit a fraction with 1 as the denominator.

Every fraction is a rational number.

$\frac{2}{3}$, $\frac{18}{7}$, $\frac{19}{11}$

Where the numerator and denominator are whole numbers and the denominator is non equal to zero.

Positive rational numbers

A rational number is positive if its numerator and denominator accept the same signs (either both positive or both negative).

$\frac{-5}{-6}$, $\frac{vii}{12}$, $\frac{-xix}{-12}$, $\frac{42}{85}$

Negative rational numbers

A rational number is negative if its numerator and denominator have opposite signs (one of them is positive and the other is negative).

$\frac{-32}{43}$, $\frac{27}{-63}$, $\frac{-12}{14}$, $\frac{9}{-16}$

Decimals: 0.45, 0.5446, 0.01864. Since decimals tin can be written equally fractions with denominator as 10, 100, g … decimal numbers also considered rational numbers.

Non-Examples of Rational Number

The square root, cube roots, etc., of natural numbers if their exact value cannot exist determined.

$\sqrt{2}$, $\sqrt{3}$, $\sqrt[2]{8}$, $\sqrt[3]{81}$, $\sqrt[4]{27}$

$\sqrt{3}$, – $\sqrt{v}$, 8 + $\sqrt{x}$, $\sqrt{2}$ + $\sqrt{3}$

Standard Form of Rational Numbers

A rational number, $\frac{p}{q}$, is said to be in standard class if the numerator and the denominator (p and q) have no common divisor (or factor) except 1 and the denominator "q " is positive.

How to convert a rational number into standard form:

Consider the rational number $\frac{xviii}{–24}$;

Let's come across how nosotros can convert this rational number into its standard form.

Pace i: Make sure that the denominator is positive. If not, multiply the numerator and the denominator by "–ane" to change the sign.

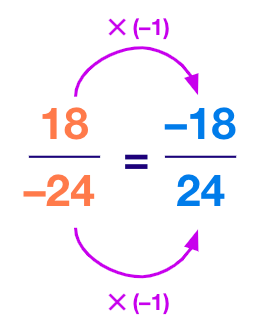
Step two: Discover all the mutual divisors of the numerator and the denominator.
Here, eighteen and 24 have common divisors 1, 2, 3, 6.

Stride iii: Dissever the numerator and the denominator past their greatest common divisor. Similar this:

Divide the numerator and the denominator by their greatest common

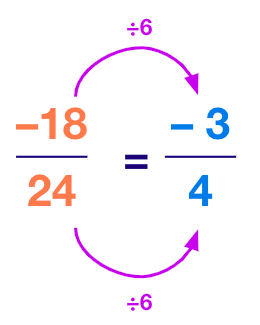
No Step three required! Equally you can see $\frac{–iii}{4}$ is a standard rational number and is the standard grade of $\frac{18}{–24}$.

Consider the decimal number 0.5

Step 1: To convert a decimal number into a standard rational number, we beginning catechumen it into a rational number.

convert a decimal number into a rational number

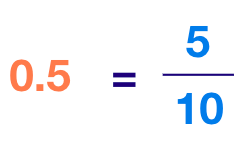
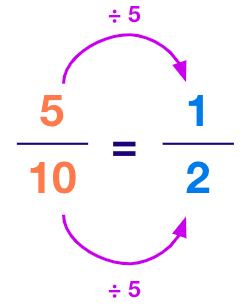
Step 2: Now follow the aforementioned steps for converting a rational number into its standard class equally divers above and we get

converting a rational number into its standard form

Operations on Rational Numbers

Addition of Rational Numbers:

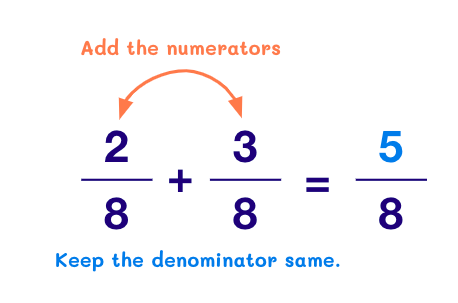
When the denominators of the given rational numbers are equal:

Add the numerators and keep the denominators the same.

For instance, we can add ii rational numbers 2/8 and ⅜ like this:

When the denominators of given numbers are diff:

For example, to add the rational numbers ⅖ and ¾

Stride i: Observe the LCM of the denominator of the given rational numbers. Here, the LCM of 4 and 5 is 20.

Pace 2: Convert each rational number into an equivalent rational number with the LCM every bit the new denominator.

Convert rational number into an equivalent rational number with the LCM

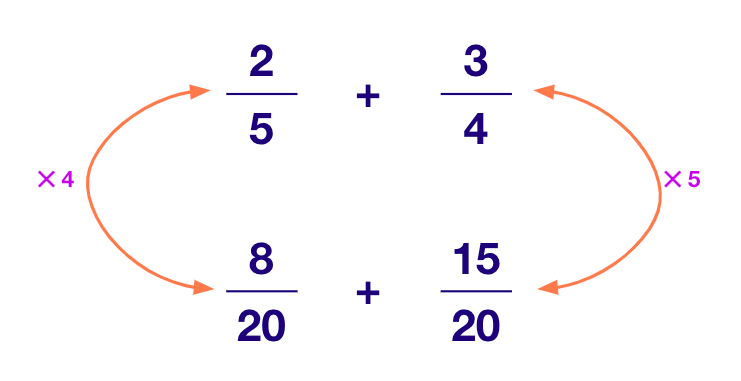
Step 3: For these new rational numbers, add the numerators and go on the denominators the same and that will exist the final answer.

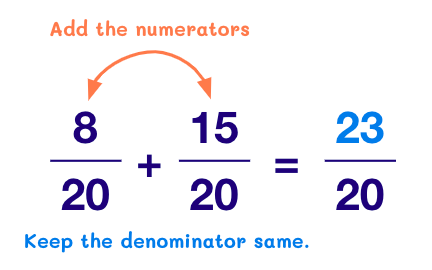
Therefore, ⅖ + ¾ = 23/20.

Subtraction of Rational Numbers:

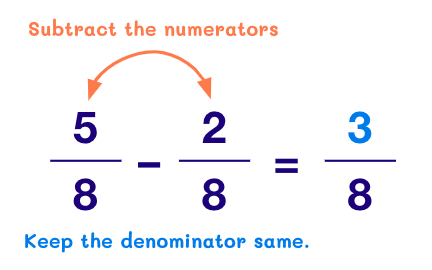
When the denominators of the given numbers are equal:

Subtract the numerators and go along the denominator the aforementioned.

For case:

Subtract the numerators and keep the denominator the same.

When the denominators of the given numbers are unequal:

For example, to subtract ⅓ from ½

Step one: Find the LCM of the denominator of the given rational numbers. In this example, the LCM of 2 and 3 is 6.

Step 2: Convert each rational number into an equivalent rational number with the LCM every bit the new denominator.

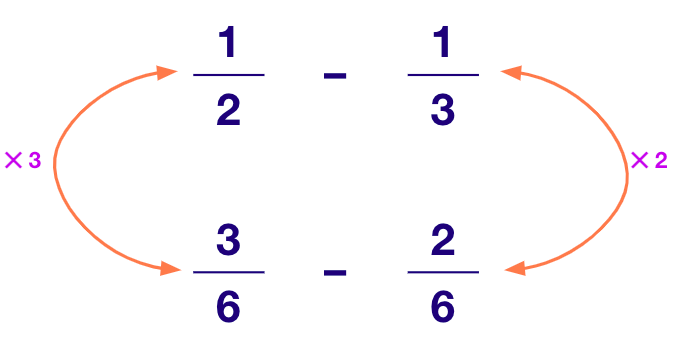
Step 3: Follow the steps of subtraction of rational numbers with equal denominators.

Therefore, ½ – ⅓ = ⅙

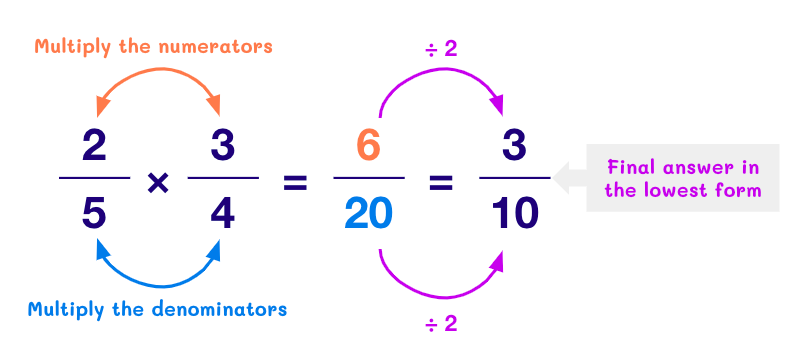
Multiplication of Rational Numbers:

This is perchance the easiest operation on rational numbers.

To multiply two rational numbers, simply multiply the numerator and denominator of the showtime rational number with the numerator and denominator of the second rational number respectively.

For example, to multiply the rational numbers ⅖ and ¾,

Solved Examples

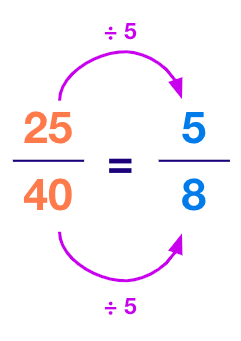
Example i: Express $\frac{25}{xl}$ in standard form.

Solution:

Greatest divisor of 25 and forty = five

Example 2: Add: $\frac{ii}{11}+\frac{7}{11}$.

Solution:

$\frac{2}{11}+\frac{7}{11}= \frac{2+7}{11}=\frac{9}{11}$

Instance three: Subtract $\frac{five}{7}$ from $\frac{nine}{seven}$.

Solution:

$\frac{9}{7}–\frac{5}{vii}= \frac{9–5}{vii}=\frac{ii}{7}$

Practice Problems

Rational Numbers

Attend this Quiz & Test your knowledge.

398/i

0/398

ane/398

398 is non a rational number.

Correct answer is: 398/1
Whole numbers can be expressed as rational numbers with denominator 1.
398 = $\frac{398}{1}$

$\frac{x}{7}$

$\frac{seven}{x}$

$\frac{0.7}{10}$

0.7 is not a rational number

Correct answer is: $\frac{7}{10}$
Removing the decimal point we can represent $\frac{7}{10}$ every bit 0.7

$\frac{2}{five}$

$\frac{-x}{3}$

$\frac{3}{-10}$

Correct answer is: $\frac{-10}{3}$
Greatest common divisor of 20 and 15 is v; so dividing both the numerator and denominator in –twenty/15 by 5 gives us –10/3.

$\frac{64}{81}$

one

$\frac{72}{81}$

Correct answer is: i
$\frac{8}{9}\times \frac{9}{8}=\frac{8×nine}{nine×eight}= \frac{72}{72}$ = 1

Frequently Asked Questions

Yeah, i is a rational number as it can be expressed in the grade of a fraction as $\frac{1}{1}$.

How practise you identify a rational number?

Whatever number that can be expressed in the form of a fraction is a rational number. Note that the denominator of a rational number cannot be 0.

How to discover the standard form of rational number?

Divide the numerator and denominator with their greatest common divisor.